Fundamentals of Electric Circuits Review

Posted on 2020-08-31 Edited on 2025-05-15 Views: Word count in article: 2.9k Reading time ≈ 10 mins.

This document is intended for the reviewing of the course Fundamentals of Electric Circuits.

Preface

Current

Current is the rate of charge flow past a given point in a given direction.

$$ i = \frac{\mathop{dq}}{\mathop{dt}} $$

Voltage

Voltage is the energy required to move 1 C of charge through an element.

$$ v = \frac{dw}{dq} $$

Power

Power is the energy supplied or absorbed per unit time.

$$ p = \frac{dw}{dt} = vi $$

Passive Sign Convention

Passive sign convention is satisfied if the direction of current is selected such that current enters through the terminal that is more positively biased.

Law of Energy Conservation

∑p = 0

Sources

An ideal voltage source has zero internal resistance and is capable of producing any amount of current.
An ideal current source has infinite resistance. It is able to generate any voltage to establish the desired current through it.
Ideal dependent source

Basic Laws

Ohm’s Law

v = Ri

i = Gv

$$ p = vi = i^2R = v^2G = \frac{v^2}{R} = \frac{i^2}{G} $$

$$ R = \rho\frac{l}{A} $$

open circuit and short circuit

Topology

A branch represents any single element in a circuit.
A node is the point of connection between two or more branches.
A loop is any closed path in a circuit.
A set of loops is said independent when any of them contains at least one new branch in addition to all others.
A mesh is a loop which does not contain any other loops within it.

b = m + n − 1

Kirchhoff’s Law

Principle of the Conservation of Charge

Kirchhoff’s Current Law (KCL)

The algebraic sum of currents entering a node (or a closed boundary) is zero. In other words, the sum of the currents entering a node is equal to that leaving the node.

$$ 0 = \frac{dq}{dt} = \sum_{n = 1}^N i_n $$

Kirchhoff’s Voltage Law (KVL)

The algebraic sum of all voltages around a loop is zero.
Sum of voltage drops = Sum of voltage rises.

$$ \sum_{m=1}^M w_m = 0 = \sum_{m=1}^M qv_m \Rightarrow \sum_{m=1}^M v_m = 0 $$

Equivalent Circuits/Sources

Two circuits (or sources) are said to be equivalent if they have the same V-I relationship at their terminals.

Resistance

$$ R_\text{eq} = \frac{R_1R_2}{R_1 + R_2}, \quad G_\text{eq} = G_1 + G_2 $$

The Principle of Voltage Division

Resistors in series

$$ v_n = \frac{R_n}{\sum_{n=1}^N R_n} v $$

The Principle of Current Division

Resistors in parallel

$$ i_n = \frac{G_n}{\sum_{n=1}^N G_n} $$

Wye-Delta Transformation

Delta to Wye

$$ \left\{ \begin{aligned} R_1 = \frac{R_bR_c}{R_a + R_b +R_c} &\\ R_2 = \frac{R_cR_a}{R_a + R_b +R_c} &\\ R_3 = \frac{R_aR_b}{R_a + R_b +R_c} &\\ \end{aligned} \right. $$

Wye to Delta

$$ \left\{ \begin{aligned} R_a = R_2 + R_3 + \frac{R_2R_3}{R_1} &\\ R_b = R_1 + R_3 + \frac{R_1R_3}{R_2} &\\ R_c = R_1 + R_2 + \frac{R_1R_2}{R_3} &\\ \end{aligned} \right. $$

Y-networks is more like a serial connection, and Δ network is more like a parallel connection.

R_Y < R_Δ

When the Y and Δ network is balanced

$$ \left\{ \begin{aligned} R_1 = R_2 = R_3 = R_Y \\ R_a = R_b = R_c = R_\Delta \end{aligned} \right.\Rightarrow\left\{ \begin{aligned} & R_Y = \frac{R_\Delta}{3}\\ & R_\Delta = 3R_Y\\ \end{aligned} \right. $$

Systematic Methods for Circuit Analysis

Nodal Voltage Analysis

Choose one node as the reference node, then label the voltage on the remaining nodes
Apply KCL to each non-reference nodes, branch currents represented in terms of node voltages according to Ohm’s Law
Solve the n − 1 simultaneous equations

Nodal analysis with voltage sources
Case 1 - the V-source connects to the reference node
Case 2 - the V-source connects to two non-reference nodes
- Treat these two nodes as one supernode

Nodal analysis is good for

The network contains many elements connected in parallel

The network contains many current sources

The circuit has fewer nodes than meshes

Node voltages are what being solved for

Mesh Current Analysis

Label the mesh currents
Apply KVL to each of the n meshes, write the voltages across each elements in terms of the mesh currents according to the Ohm’s Law
Solve the n simultaneous equations

Always consider the circuit in clockwise direction

Mesh analysis with current sources
Case 1 - the current source is NOT shared by multiple meshes
Case 2 - the current source IS shared by multiple meshes
- Treat the two sharing meshes as one supermesh

Mesh analysis is applicable for planar circuits Mesh analysis is good for

The network contains many serially connected elements

The network contains many voltage sources

The circuit has fewer meshes than nodes

Branch or mesh currents are what being solved for

Circuit Theorems

Linear Circuits

HomogeneityProperty

f(αx) = αf(x) = αy

Additivity Property

$$ \left\{\begin{aligned} y_1 = f(x_1) \\ y_2 = f(x_2) \end{aligned}\right.\Rightarrow f(x_1 + x_2) = y_1 + y_2 $$

Linearity

f(αx₁+βx₂) = αy₁ + βy₂

Superposition Theorem

In a linear system, the voltage across (or current through) an element is the algebraic sum of those caused by each independent source acting alone

Turn off all independent source except one, find the output
Repeat Step 1 for other sources
Adding up

Source Transformation

two circuits are equivalent if their voltage versus current (v-i) relations at port are identical

Thevenin’s Theorem

A linear two terminal circuit can be replaced by an equivalent circuit consisting of a voltage source V_Th in series with a resistor R_Th .

Open the circuit, to find out the voltage between terminals
Add an assumed source (generally voltage source), then calculate the equivalent resistance

Maximum Power Transfer

$$ R_L = R_\text{Th},\quad p_\text{max} =\frac{V_\text{Th}^2}{4R_\text{Th}} $$

Norton’s Theorem

A linear two terminal circuit can be replaced by an equivalent circuit consisting of a current source I_N and a parallel > resistor R_N

Find the open-circuit voltage
Find the short-circuit current
The equivalent or input resistance R_in at terminals when all independent sources are turned off

Operational Amplifiers

Basics of Op-Amp

Op amp is an active electric element

input resistance
output resistance
open loop voltage gain

Ideal op amp

R_o ≈ 0

R_i = ∞ ⇒ i₁ ≈ 0, i₂ ≈ 0

A ≈ ∞ ⇒ v₁ ≈ v₂

virtual opening and virtual shorting

Inverting Amplifier

$$ v_0 = -\frac{R_f}{R_1}v_i $$

Trans-Resistance Amplifier

$$ \frac{v_o}{i_s} = -R $$

Summing Amplifier/Summer

$$ v_0 = -\left( \frac{R_f}{R_1}v_1 + \frac{R_f}{R_2}v_2 + \frac{R_f}{R_3}v_3 \right) $$

Non-Inverting Amplifier

$$ A_v = \frac{v_o}{v_i} = 1 + \frac{R_f}{R_1} $$

Voltage Follower

Also named unity gain amplifier

Difference Amplifier

$$ v_o = \frac{R_2(1 + R_1/R_2)}{R_1(1+R_3/R_4)}v_2 - \frac{R_2}{R_1}v_1 $$

If R₁ = R₂, R₃ = R₄, this becomes a subtractor

Capacitors and Inductors

Capacitor

q = Cv

For planar capacitor

$$ C = \frac{\epsilon A}{d} $$

$$ v(t) = \frac{1}{C}\int_{-\infty}^t i(\tau)\mathop{d\tau} + v(t_0) $$

$$ i = \frac{dq}{dt} = C\frac{dv}{dt} $$

$$ w = \frac{1}{2}Cv^2 = \frac{q^2}{2C} $$

Capacitor is open circuit to dc
Voltage on a capacitor must be continuous
In parallel: C_eq = C₁ + C₂ + ⋯ + C_N
In series: $\frac{1}{C_{eq}} = \frac{1}{C_1} + \frac{1}{C_2} + \cdots + \frac{1}{C_N}$

Inductor

$$ v = L\frac{di}{dt} $$

For solenoid

$$ L = \frac{N^2\mu A}{l} $$

$$ i(t) = \frac{1}{L}\int_{-\infty}^t v(\tau)\mathop{d\tau} + i(t_0) $$

Inductor is short circuit to dc
Current on a inductor must be continuous
In parallel: $\frac{1}{L_{eq}} = \frac{1}{L_1} + \frac{1}{L_2} + \cdots + \frac{1}{L_N}$
In series: L_eq = L₁ + L₂ + ⋯ + L_N

Singularity Functions

Step Function

Impulse/Delta Function

∫_0⁻^0⁺δ(t)dt = 1

Unit Ramp Function

Relationship

In form of differentiation

$$ \delta(t) = \frac{du(t)}{dt},\quad u(t) = \frac{dr(t)}{dt} $$

In form of integration

u(t) = ∫_− ∞^tδ(λ)dλ , r(t) = ∫_− ∞^tu(λ)dλ

First Order Circuits

Source-Free RC Circuit

v(t) = V₀e^− t/RC = V₀e^− t/τ

natural response
decay rate

$$ w_R(t) = \frac{1}{2}CV_0^2 \left(1-e^{-2t/\tau}\right) $$

initial voltage
time constant

Source-Free RL Circuit

$$ i(t) = i(0)e^{-Rt/L} = i(0)e^{-t/\tau},\quad \tau = \frac{L}{R} $$

$$ w_R(t) = \frac{1}{2}LI_0^2 \left(1-e^{-2t/\tau}\right) $$

initial current
time constant

Step Response of RC Circuit

Complete/Total Response

$$ v(t) = \left\{\begin{aligned} & V_0, & t < 0 \\ & V_s + (V_0 - V_s)e^{-t/\tau}, & t > 0 \end{aligned}\right.,\quad \tau = RC $$

natural response
forced response

transient response
steady-state response

Step Response of RL Circuit

$$ i(t) = \left\{\begin{aligned} & I_0, & t < 0 \\ & \frac{V_s}{R} + \left(I_0 - \frac{V_s}{R}\right)e^{-t/\tau}, & t > 0 \end{aligned}\right.,\quad \tau = \frac{L}{R} $$

Instantaneous value = Final + (Initial−Final)e^{− (t−t₀)/τ}

Second Order Circuits

Source-Free Series RLC Circuits

$$ Ri + L\frac{di}{dt} + \frac{1}{C}\int_{-\infty}^t i(\tau)\mathop{d\tau} = 0 \Rightarrow \frac{d^2i}{dt^2} + \frac{R}{L}\frac{di}{dt} + \frac{i}{LC} = 0 $$

The solution is in the form of

i(t) = A₁e^s₁t + A₂e^s₂t

In which

$$ \begin{aligned} & \Rightarrow s^2 + \frac{R}{L}s + \frac{1}{LC} = 0 \\ & \Rightarrow s_1 = -\alpha + \sqrt{\alpha^2 - \omega_0^2}, \quad s_2 = -\alpha - \sqrt{\alpha^2 - \omega_0^2} \end{aligned} $$

Where α = R/2L is damping factor, $\omega_0 = 1/\sqrt{LC}$ is resonant frequency.

over damped, α > ω₀
critically damped, α = ω₀
under damped, α < ω₀
undamped, α = 0

Source-Free Parallel RLC Circuits

$$ \frac{v}{R} + \frac{1}{L}\int_{-\infty}^t v(\tau)\mathop{d\tau} + C\frac{dv}{dt} = 0 \Rightarrow \frac{d^2v}{dt^2} + \frac{1}{RC}\frac{dv}{dt} + \frac{1}{LC}v = 0 $$

The general solution is

v(t) = A₁e^s₁t + A₂e^s₂t

Where

$$ \alpha = \frac{1}{2RC},\quad \omega_0 = \frac{1}{\sqrt{LC}} $$

Step Response of Series RLC Circuit

Over damped - v(t) = V_s + A₁e^s₁t + A₂e^s₂t
Critically damped - v(t) = V_s + (A₁+A₂t)r^− αt
Under damped - v(t) = V_s + (A₁cosω_dt+A₂sinω_dt)e^− αt

$$ \alpha = \frac{R}{2L} $$

Step Response pf Parallel RLC Circuit

Over damped - i(t) = I_s + A₁e^s₁t + A₂e^s₂t
Critically damped - i(t) = I_s + (A₁+A₂t)r^− αt
Under damped - i(t) = I_s + (A₁cosω_dt+A₂sinω_dt)e^− αt

$$ \alpha = \frac{1}{2RC} $$

Sinusoids and Phasors

Sinusoids

v(t) = V_msin (ωt+ϕ)

amplitude
angular frequency
argument
phase
period
leads
lags
out of phase
in phase
anti phase

Complex Number

rectangular form z = x + jy
polar form z = r∠ϕ
exponential form z = re^jϕ

Where $r = \sqrt{x^2 + y^2}$ is the modulus, $\phi = \tan^{-1}\frac{y}{x}$ is the argument.

multiplication

z₁z₂ = r₁r₂∠(ϕ₁+ϕ₂)

division

$$ \frac{z_1}{z_2} = \frac{r_1}{r_2}\angle(\phi_1 - \phi_2) $$

reciprocal

$$ \frac{1}{z} = \frac{1}{r}\angle(-\phi) $$

square root

$$ \sqrt{z} = \sqrt{r}\angle(\phi/2) $$

complex conjugate

z^* = x − jy = r∠(−ϕ) = re^− jϕ

Phasors

v(t) = V_mcos (ωt+ϕ) = ℜ[V_me^j(ωt+ϕ)]

Where V = V_me^jϕ = V_m∠ϕ is the phasor

Time Domain	Phasor Domain
dv /dt	jωV
∫vdt	V/jω

Generalized Ohm’s Law

V = RI

V = jωLI

I = jωCV

resistance
reactance
impedance

Z + R + jX

admittance

Element	Impedance
R	Z = R
L	Z = jωL
C	$\mathbf{Z} = \frac{1}{j\omega C}$

Ohm’s Law

V = ZI

Sinusoid Steady State Analysis

To obtain superposition theorem, the sources in the circuit must have the same frequency

AC Power Analysis

Instantaneous Power

$$ \begin{aligned} p(t) & = v(t)i(t) \\ & = V_mI_m\cos(\omega t + \theta_v)\cos(\omega t + v_i) \\ & = \frac{1}{2}V_mI_m\cos(2\omega t + \theta_v + \theta_i) \end{aligned} $$

Average Power

effective value / RMS value

$$ P = \frac{1}{2}V_mI_m\cos(\theta_v - \theta_i) $$

apparent power S
power factor
power factor angle
complex power

$$ \mathbf{S} = \frac{1}{2}\mathbf{V}\mathbf{I}^*= \mathbf{V}_\text{rms}\mathbf{I}^*_\text{rms} $$

real power
reactive / quadrature power

P = ℜ(S), Q = ℑ(S)

Maximum Average Power Transfer

$$ P_\text{max} = \frac{|\mathbf{V}_\text{Th}|^2}{8R_\text{Th}} $$

Magnetically Coupled Circuits

Faraday’s Law pf electromagnetic induction

$$ e = N\frac{d\phi}{dt} $$

Self Inductance

self inductance

$$ v = N\frac{d\phi}{dt} = N\frac{d\phi}{di}\frac{di}{dt} = L\frac{di}{dt} $$

$$ L = N\frac{d\phi}{di_L} $$

Mutual Inductance

Dot Convention

If a current enters the dotted terminal of one coil, the reference polarity of the mutual voltage in the second coil is positive at the dotted terminal of the second coil.

Cascade Connection

Series aiding connection

L = L₁ + L₂ + 2M

Else opposing connected, should be minus 2M

Coefficient of Coupling

$$ k = \frac{\phi_{12}}{\phi_1} = \frac{\phi_{12}}{\phi_{11} + \phi_{12}} = \frac{M}{\sqrt{L_1L_2}} $$

$$ M = k\sqrt{L_1L_2} $$

Transformers

Linear / Air-Core Transformers

input impedance
reflected / coupled impedance

$$ \mathbf{Z}_\text{R} = \frac{\omega^2M^2}{R_2 + j\omega L_2 + \mathbf{Z}_L} $$

port V-I property

$$ \begin{bmatrix} \mathbf{V}_1 \\ \mathbf{V_2} \end{bmatrix} = \begin{bmatrix} j\omega L_1 & j\omega M \\ j\omega M & j\omega L_2 \end{bmatrix} = \begin{bmatrix} \mathbf{I}_1 \\ \mathbf{I_2} \end{bmatrix} $$

Ideal Transformers

$$ \frac{V_2}{V_1} = \frac{N_2}{N_1} $$

$$ \mathbf{Z}_\text{in} = \frac{\mathbf{Z}_L}{n^2} $$

Frequency Response

Y(ω) = H(ω)X(ω)

Laplace Transform

one-side / unilateral Laplace Transform

ℒ[f(t)] = F(s) = ∫_0⁻^∞f(t)e^− stdt

Where

s = σ + jω

$$ \mathscr{L}^{-1}[F(s)] = f(t) = \frac{1}{2\pi j}\int_{\sigma - j\infty}^{\sigma + j\infty} F(s)e^{st}\mathop{ds} $$

Properties

Linearity

ℒ[a₁f₁(t)+a₂f₂(t)] = a₁F₁(t) + a₂F₂(t)

Scale changing

$$ \mathscr{L}[f(at)] = \frac{1}{a}F\left(\frac{s}{a}\right),\quad a > 0 $$

Time shift

ℒ[f(t−a)u(t−a)] = e^− asF(s), a > 0

Frequency shift

ℒ[e^− atf(t)] = F(s+a)

Differentiation in time domain

$$ \mathscr{L}\left[\frac{df(t)}{dt}\right] = sF(s) - f(0^-) $$

Initial value theorem

f(0⁺) = lim_{s → ∞}sF(s)

Final value theorem

f(∞) = lim_s → 0sF(s)

Higher order differentiation in time domain

$$ \mathscr{L}\left[\frac{d^nf(t)}{dt^n}\right] = s^nF(s) - s^{n-1}f(0^-) - s^{n-2}\frac{df(0^-)}{dt} - \cdots - \frac{d^{n-1}f(0^-)}{d^{n-1}} $$

Integration in time domain

$$ \mathscr{L}\left[\int_{0^-}^t f(x)\mathop{dx}\right] = \frac{F(s)}{s} $$

Differentiation in frequency domain

$$ \mathscr{L}[t^nf(t)] = (-1)^n\frac{d^nF(s)}{ds^n} $$

…

Method of Partial Expansion

Residue Method

Case 1: Simple distinct poles

$$ F(s) = \frac{N(s)}{(s+p_1)(s+p_2)\cdots(s+p_n)} $$

$$ \Rightarrow F(s) = \frac{k_1}{s + p_1} + \frac{k_1}{s + p_2} + \cdots + \frac{k_1}{s + p_n} $$

Where k_i are known as residuals

By Heaviside’s Theorem

k_i = (s+p_i)F(s)|_{s = − p_i}

Case 2: Repeated poles

…

Method of Algebra

Solving Equations at Specific Values

Inverse Laplace Transform

Convolution Integral

x(t) = ∫_− ∞^∞x(λ)δ(t−λ)dλ = x(t) * h(t)

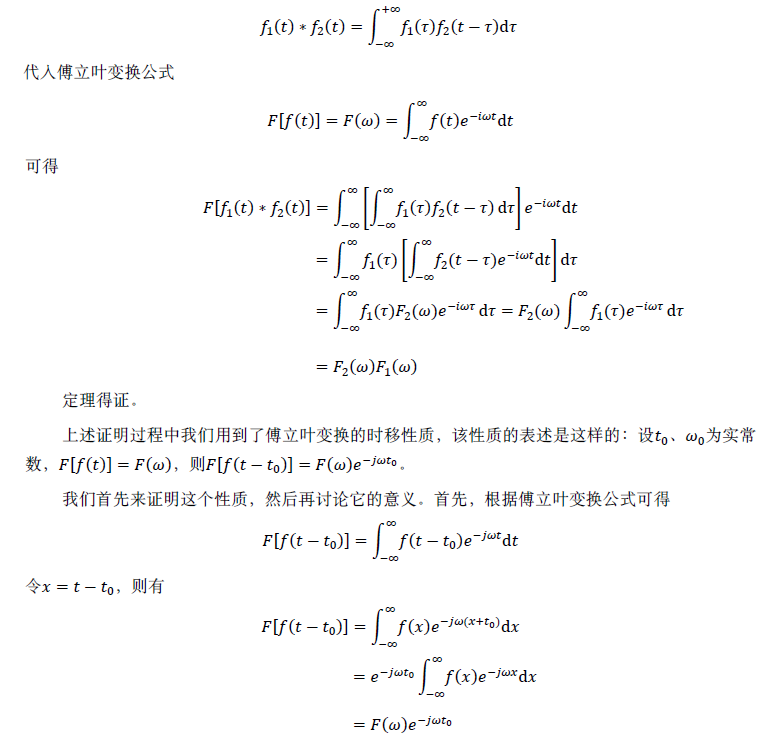
Circuit Analysis in s-Domain

Transform the circuit from the time domain to the s-domain
Solve the circuit using nodal analysis, mesh analysis, etc.
Take the inverse transform of the solution and thus obtain the solution in the time domain

inductor

$$ V(s) = sLI - Li(0^-), \quad I(s) = \frac{1}{sL}V(s) + \frac{i(0^-)}{s} $$

capacitor

$$ V(s) = \frac{1}{sC}I(s) + \frac{v(0^-)}{s}, \quad I(s) = C[sV - v(0^-)] $$

impedance

Resistor	Inductor	Capacitor
Z(s) = R	Z(s) = sL	Z(s) = 1/sC